Unit-2 Look Angle Determination.ppt inSC
Download as PPT, PDF0 likes24 views
This concept determines the look angle concept in satellite communication.
1 of 25
Download to read offline
























Recommended
GIS_lec 2_Different_Spatial coordinate system.ppt



GIS_lec 2_Different_Spatial coordinate system.pptMahinMobarrat
╠²
Coordinate System: understanding the different kind of co-ordinate systems used for representing the exact place in earth. Surveying ii ajith sir class 2,3,4



Surveying ii ajith sir class 2,3,4SHAMJITH KM
╠²
A graticule is a network of lines, sometimes described as a grid, that is used for geographic plotting, scale, and focusing depending on the application. A common example is a grid of lines on a map corresponding to longitude and latitude.Combined gis 2(GEOGRAPHIC INFORMATION SYSTEM)



Combined gis 2(GEOGRAPHIC INFORMATION SYSTEM)musadoto
╠²
Distortions
ŌĆó The earth is spherical, and a simple way of mapping it without distortion is to map it on a globe. However, mapping on globes is not possible.
ŌĆó The transformation from the three-dimensional ellipsoid/sphere to the two-dimensional plane (flat) surface is not possible without some form of distortion.
ŌĆó The distortions increase as the distance from the central point of the projection increases
ŌĆó Areas smaller than 25 x 25 km:
No distortions
ŌĆó Areas larger than 25 x 25 km:
Always distortions
ŌĆó Map projections are used to control/minimize the distortions
Classification and properties of map projections
Properties of map projections
ŌĆó Areas are everywhere correctly represented
ŌĆó All distances are correctly represented
ŌĆó All directions on the map are the same as on Earth
ŌĆó All angles are correctly represented
ŌĆó The shape of any area is correctly represented (e.g. a circle projected would remain a circle)Aerial NAVIGATION and FLIGHT PLANNING.pptx



Aerial NAVIGATION and FLIGHT PLANNING.pptxEhtasham Amir
╠²
Air navigation for BS aviation
undergraduate programme . This ppt explains basic concepts of air navigation. this is used to teach BS aviation, undergrad datum



datumRiya Gupta
╠²
Spheroid, datum, projection, and coordinate systems are used to locate positions on Earth. A spheroid is a mathematical model that approximates the Earth's shape as an oblate spheroid. A datum defines the reference frame for latitude and longitude coordinates and relates the spheroid to the Earth's center. Projections transform 3D spheroid coordinates onto a 2D surface like a map, introducing some distortion of shapes, areas, distances or directions. Common projections include transverse Mercator, UTM, and lambert conformal conic. Coordinate systems then allow measurement of positions on the projected 2D surface. Understanding these concepts is important for accurately locating geographic features.Map Projections



Map ProjectionsJohn Reiser
╠²
This document discusses different types of map projections used to represent the spherical earth on a flat surface. It describes how all projections involve some distortion of properties like shapes, areas, distances or directions. The key types are conformal, equivalent, and equidistant projections. It explains the concepts of projection surfaces like cones, cylinders and planes, as well as variables like the light source and orientation. Specific common projections are also outlined, such as Mercator, Lambert conformal conic, and azimuthal equidistant, along with their characteristic distortions and uses.projections.ppt



projections.pptGauravSingh338805
╠²
A map projection is a method for mapping spatial patterns on a curved surface (the Earth's surface) to a flat surface. Projections always introduce some distortion of properties like shape, area, direction, or distance. Common projections include Albers, Lambert Azimuthal Equal Area, Mercator, Miller, Mollweide, and Orthographic. Coordinate systems like State Plane and UTM provide a measurement framework by assigning coordinates using projections. Datums define a reference system to place coordinate systems on the Earth's surface. Projecting data between different coordinate systems and datums allows spatial data sets that were originally incompatible to be made compatible.projections.ppt



projections.pptssuser37f552
╠²
A map projection is a method for mapping spatial patterns on a curved surface (the Earth's surface) to a flat surface. Projections always introduce some distortion of properties like shape, area, direction, or distance. Common projections include Albers, Lambert Azimuthal Equal Area, Mercator, Miller, Mollweide, and Orthographic. Coordinate systems like State Plane and UTM provide a measurement framework by assigning coordinates using projections. Datums define a reference system to place coordinate systems on the Earth's surface and commonly used datums include NAD27, NAD83, and WGS84. Projecting spatial datasets allows data from different sources and projections to be made compatible for analysis.projections.ppt



projections.pptssuser37f552
╠²
A map projection is a method for mapping spatial patterns on a curved surface (the Earth's surface) to a flat surface. Projections always introduce some distortion of properties like shape, area, direction, or distance. Common projections include Albers, Lambert Azimuthal Equal Area, Mercator, Miller, Mollweide, and Orthographic. Coordinate systems like State Plane and UTM provide a measurement framework by assigning coordinates using projections. Datums define a reference system to place coordinate systems on the Earth's surface and commonly used datums include NAD27, NAD83, and WGS84. Projecting spatial datasets allows data from different sources and projections to be made compatible for analysis.Orbital mechanics



Orbital mechanicsRAVIKIRAN ANANDE
╠²
1. Kepler's laws of planetary motion describe the motion of planets and satellites in orbit. The orbital period is determined by the semimajor axis of the elliptical orbit.
2. A geostationary orbit is circular, at an altitude that matches the orbital period to Earth's sidereal day, and in the equatorial plane. A geosynchronous orbit has the correct period but may have eccentricity or inclination.
3. Orbital elements like eccentricity, semimajor axis, inclination and nodes define the characteristics of Earth-orbiting satellites. Perturbations from factors like the Sun and Moon cause the orbital elements and position to change over time.Earth Coordinate Systems 



Earth Coordinate Systems Sheikh Maryam
╠²
This Presentation is to made concepts about measuring the earth (to locate position of any person on the whole earth). For this purpose we re going step by step basis in this presentation.These steps are mentioned as contents. After that you may able to learn about measuring a person's position of earth. Thank you!
Map projection



Map projectionkaslinsas
╠²
This document discusses different types of map projections. It begins by defining map projection as a systematic drawing of parallels and meridians on a plane surface that corresponds to locations on Earth. It notes that all projections necessarily distort the surface in some way. Projections are classified based on their construction method, development surface, preserved properties, and position of the light source. Common projection types discussed include cylindrical, conic, azimuthal, Mercator, sinusoidal, and polyconic. The key properties and uses of each type are outlined. The document emphasizes that the purpose of the map determines the best projection to use.projection



projectionPreeti Tiwari
╠²
Projections are an essentials part of every datasets. Basically, a projection is the mathematical operation needed to go from the planet actual shape to a flat map according to the Geographic Coordinate System.Map projection



Map projectionSumant Diwakar
╠²
Map projections convert latitude and longitude coordinates on a spherical Earth into two-dimensional planar coordinates by applying a mathematical transformation. They define a coordinate system and allow measurement of horizontal and vertical distances to establish positions of geographic features. Creating a projection involves selecting an Earth model (sphere or ellipsoid) and transforming geographic to planar coordinates. Common projections preserve either shapes, areas, distances or directions depending on the mapping needs.Geodetic systems



Geodetic systemsMoses Robby
╠²
The document discusses coordinate systems used in geodesy. It describes how a point on Earth's surface is projected onto a reference spheroid and geoid to define its horizontal and vertical positions. Geodetic coordinates use latitude, longitude, and elevation to precisely locate points on the spheroid. It also describes the Cartesian coordinate system and WGS 84 system used by the U.S. Defense Department as a geocentric reference frame. Key elements like great circles, parallels, and meridians are defined on the spheroid for determining latitude and longitude.Field Astronomy



Field AstronomyMujeeb Muji
╠²
This document provides an overview of field astronomy concepts. It defines key celestial coordinate systems used to specify the position of heavenly bodies, including the horizon system (using altitude and azimuth), independent equatorial system (using right ascension and declination), and dependent equatorial system (using declination and hour angle). It also describes the celestial latitude and longitude system. Spherical trigonometry formulas are presented for computing angles and distances on the celestial sphere. The astronomical triangle relating altitude, declination, and latitude is illustrated. Key terms like latitude, longitude, declination, and right ascension are defined.Ground track of satellite and factors affecting it



Ground track of satellite and factors affecting itRabi Shrestha
╠²
Ground track of satellite, and the major factors affecting it are highlighted in the slide.Theodolite surveying



Theodolite surveyingPhurba Tamang
╠²
The document provides information about theodolite surveying including:
1. A theodolite is an instrument used to measure horizontal and vertical angles which can also be used to prolong lines, measure distances indirectly, and for leveling.
2. Theodolite traversing involves establishing control points by measuring angles and distances between traverse stations to calculate positions.
3. Components of a theodolite include a telescope that can rotate vertically and a compass to determine direction, along with accessories like a tripod, rods, and tapes used in surveying.Basic Civil and Mechanical Engineering UNIT II PPT.pptx



Basic Civil and Mechanical Engineering UNIT II PPT.pptxSombabu Yadlapalli
╠²
Surveying: Concept of Surveying ŌĆō Classification of Surveying based on different criteriaŌĆō Fundamental principles in Surveying -Units and conversions -Angular Measurements & Levelling -Simple problems (Height of instrument Method) - Contour-Uses of contour mapping
Geotechnical Engineering: Origin of soil ŌĆōSoil formationŌĆō Types of soils ŌĆō Grain size analysis ŌĆō I.S Classification ŌĆō Visual classification - Site investigation & Type foundations
FIELD ASTRONOMY SURVEYING



FIELD ASTRONOMY SURVEYINGKavin Raval
╠²
This document provides information about different coordinate systems used in astronomy and mapping. It discusses the celestial sphere projection and altitude-azimuth and equatorial coordinate systems used to describe positions of celestial objects. It also covers horizon coordinates, celestial coordinates including right ascension and declination, and concepts like the celestial equator, ecliptic, and seasons. The document further summarizes coordinate systems used for mapping earth resources, including local geographic coordinates, projected coordinate systems, and specific projections like Lambert conformal conic and transverse Mercator.Satellite Communication Lec4, Chat Rooms GupshupCorner.com Pakistan.



Satellite Communication Lec4, Chat Rooms GupshupCorner.com Pakistan.Saira Honey
╠²
Satellite Communication Lecture 3 , by Chat Rooms | pakistani Chat Rooms http://www.gupshupcorner.com Chat Rooms Without Registration.Earths dimension ppt np1



Earths dimension ppt np1West Hollow MS Ms. Gill
╠²
This document provides information about Earth's four spheres: the geosphere, atmosphere, hydrosphere, and biosphere. It defines each sphere and provides some key details about their composition and interactions. The geosphere is the solid Earth, consisting of all interior layers from the surface to the core. The atmosphere is the gaseous envelope surrounding Earth. The hydrosphere includes all liquid and solid water on Earth, such as oceans, lakes, rivers, and glaciers. Finally, the biosphere comprises all living things on Earth, from microorganisms to plants and animals. The document also notes that the spheres interact and influence each other.Time Presentation 



Time Presentation Vj NiroSh
╠²
This document discusses different types of time measurement:
- Sidereal time is based on the motion of stars, while solar time is based on the motion of the sun.
- Local sidereal time is the hour angle of the first point of Aries measured from the local meridian.
- Mean solar time is measured based on the interval between the mean sun's position at the vernal equinox.
- Greenwich mean time is measured from the Greenwich meridian and is also known as universal time. Standard time uses the same time zone to avoid confusion caused by different local times.projection.pptx



projection.pptxAsmitaKanav1
╠²
The document provides an overview of map projections, which are mathematical transformations that convert the spherical coordinates on the Earth's surface to planar coordinates that can be represented on a map. It discusses the different types of projections, including cylindrical, conical, and planar projections. It also covers important concepts like datums, ellipsoids, and how different projections preserve properties like shapes, distances, areas, or directions to varying degrees based on their mathematical properties and construction.Cartography Unit_ 3.pptx



Cartography Unit_ 3.pptxwarkisafile1
╠²
This document provides information on map projections. It defines map projection as a systematic transformation of locations on Earth onto a plane. It discusses the three main types of projections: planar, cylindrical, and conic. Planar projections center on a point and are accurate near the center, cylindrical projections are rolled onto a cylinder and accurate along the equator, and conic projections use a cone and are suited to limited east-west areas near the equator. It also discusses properties like shape, area, direction, and distance distortions that occur in projections and notes no projection is perfect. Common projections like Mercator, UTM, and Robinson are described.Lecture 2.pptx.pdf



Lecture 2.pptx.pdfssuser7386811
╠²
This document provides an overview of satellite communications and orbital mechanics. It discusses the key forces that act on satellites in stable orbits, including centrifugal force and gravitational attraction. Kepler's laws of planetary motion are also summarized. The document outlines different types of satellite orbits, orbital parameters, and how to calculate look angles to track a satellite's position from an earth station. It describes the general architecture of satellite communication systems, including the space segment consisting of the satellite and ground control station, as well as different types of ground terminals.Surveying geodesy ajith sir



Surveying geodesy ajith sirSHAMJITH KM
╠²
The Earth is not a perfect sphere, but is slightly flattened at the poles. The Earth rotates daily on its tilted axis, causing seasons and influencing climate. Parallels of latitude and meridians of longitude form a grid system to locate positions on the Earth's surface. The Earth revolves around the Sun annually in an elliptical orbit, with the seasons resulting from the tilt of its axis of rotation. Precise geodetic coordinates define locations on the reference ellipsoid used to model the oblate spheroid shape of the Earth.3.2.2.a_orbitmechmodeling.pptx



3.2.2.a_orbitmechmodeling.pptxsanthyamuthu1
╠²
Orbital mechanics describes the motion of objects in orbit around other celestial bodies under the influence of gravity. An orbit is a regular, repeating path determined by Kepler's laws of planetary motion. Orbital elements such as eccentricity, semi-major axis, inclination, and others are used to quantitatively describe the size, shape, and orientation of orbits in space. Different types of orbits like low Earth orbit, geostationary orbit, and Molniya orbit are suited for different purposes like Earth observation, communications, and coverage of high latitude regions.More Related Content
Similar to Unit-2 Look Angle Determination.ppt inSC (20)
projections.ppt



projections.pptssuser37f552
╠²
A map projection is a method for mapping spatial patterns on a curved surface (the Earth's surface) to a flat surface. Projections always introduce some distortion of properties like shape, area, direction, or distance. Common projections include Albers, Lambert Azimuthal Equal Area, Mercator, Miller, Mollweide, and Orthographic. Coordinate systems like State Plane and UTM provide a measurement framework by assigning coordinates using projections. Datums define a reference system to place coordinate systems on the Earth's surface and commonly used datums include NAD27, NAD83, and WGS84. Projecting spatial datasets allows data from different sources and projections to be made compatible for analysis.Orbital mechanics



Orbital mechanicsRAVIKIRAN ANANDE
╠²
1. Kepler's laws of planetary motion describe the motion of planets and satellites in orbit. The orbital period is determined by the semimajor axis of the elliptical orbit.
2. A geostationary orbit is circular, at an altitude that matches the orbital period to Earth's sidereal day, and in the equatorial plane. A geosynchronous orbit has the correct period but may have eccentricity or inclination.
3. Orbital elements like eccentricity, semimajor axis, inclination and nodes define the characteristics of Earth-orbiting satellites. Perturbations from factors like the Sun and Moon cause the orbital elements and position to change over time.Earth Coordinate Systems 



Earth Coordinate Systems Sheikh Maryam
╠²
This Presentation is to made concepts about measuring the earth (to locate position of any person on the whole earth). For this purpose we re going step by step basis in this presentation.These steps are mentioned as contents. After that you may able to learn about measuring a person's position of earth. Thank you!
Map projection



Map projectionkaslinsas
╠²
This document discusses different types of map projections. It begins by defining map projection as a systematic drawing of parallels and meridians on a plane surface that corresponds to locations on Earth. It notes that all projections necessarily distort the surface in some way. Projections are classified based on their construction method, development surface, preserved properties, and position of the light source. Common projection types discussed include cylindrical, conic, azimuthal, Mercator, sinusoidal, and polyconic. The key properties and uses of each type are outlined. The document emphasizes that the purpose of the map determines the best projection to use.projection



projectionPreeti Tiwari
╠²
Projections are an essentials part of every datasets. Basically, a projection is the mathematical operation needed to go from the planet actual shape to a flat map according to the Geographic Coordinate System.Map projection



Map projectionSumant Diwakar
╠²
Map projections convert latitude and longitude coordinates on a spherical Earth into two-dimensional planar coordinates by applying a mathematical transformation. They define a coordinate system and allow measurement of horizontal and vertical distances to establish positions of geographic features. Creating a projection involves selecting an Earth model (sphere or ellipsoid) and transforming geographic to planar coordinates. Common projections preserve either shapes, areas, distances or directions depending on the mapping needs.Geodetic systems



Geodetic systemsMoses Robby
╠²
The document discusses coordinate systems used in geodesy. It describes how a point on Earth's surface is projected onto a reference spheroid and geoid to define its horizontal and vertical positions. Geodetic coordinates use latitude, longitude, and elevation to precisely locate points on the spheroid. It also describes the Cartesian coordinate system and WGS 84 system used by the U.S. Defense Department as a geocentric reference frame. Key elements like great circles, parallels, and meridians are defined on the spheroid for determining latitude and longitude.Field Astronomy



Field AstronomyMujeeb Muji
╠²
This document provides an overview of field astronomy concepts. It defines key celestial coordinate systems used to specify the position of heavenly bodies, including the horizon system (using altitude and azimuth), independent equatorial system (using right ascension and declination), and dependent equatorial system (using declination and hour angle). It also describes the celestial latitude and longitude system. Spherical trigonometry formulas are presented for computing angles and distances on the celestial sphere. The astronomical triangle relating altitude, declination, and latitude is illustrated. Key terms like latitude, longitude, declination, and right ascension are defined.Ground track of satellite and factors affecting it



Ground track of satellite and factors affecting itRabi Shrestha
╠²
Ground track of satellite, and the major factors affecting it are highlighted in the slide.Theodolite surveying



Theodolite surveyingPhurba Tamang
╠²
The document provides information about theodolite surveying including:
1. A theodolite is an instrument used to measure horizontal and vertical angles which can also be used to prolong lines, measure distances indirectly, and for leveling.
2. Theodolite traversing involves establishing control points by measuring angles and distances between traverse stations to calculate positions.
3. Components of a theodolite include a telescope that can rotate vertically and a compass to determine direction, along with accessories like a tripod, rods, and tapes used in surveying.Basic Civil and Mechanical Engineering UNIT II PPT.pptx



Basic Civil and Mechanical Engineering UNIT II PPT.pptxSombabu Yadlapalli
╠²
Surveying: Concept of Surveying ŌĆō Classification of Surveying based on different criteriaŌĆō Fundamental principles in Surveying -Units and conversions -Angular Measurements & Levelling -Simple problems (Height of instrument Method) - Contour-Uses of contour mapping
Geotechnical Engineering: Origin of soil ŌĆōSoil formationŌĆō Types of soils ŌĆō Grain size analysis ŌĆō I.S Classification ŌĆō Visual classification - Site investigation & Type foundations
FIELD ASTRONOMY SURVEYING



FIELD ASTRONOMY SURVEYINGKavin Raval
╠²
This document provides information about different coordinate systems used in astronomy and mapping. It discusses the celestial sphere projection and altitude-azimuth and equatorial coordinate systems used to describe positions of celestial objects. It also covers horizon coordinates, celestial coordinates including right ascension and declination, and concepts like the celestial equator, ecliptic, and seasons. The document further summarizes coordinate systems used for mapping earth resources, including local geographic coordinates, projected coordinate systems, and specific projections like Lambert conformal conic and transverse Mercator.Satellite Communication Lec4, Chat Rooms GupshupCorner.com Pakistan.



Satellite Communication Lec4, Chat Rooms GupshupCorner.com Pakistan.Saira Honey
╠²
Satellite Communication Lecture 3 , by Chat Rooms | pakistani Chat Rooms http://www.gupshupcorner.com Chat Rooms Without Registration.Earths dimension ppt np1



Earths dimension ppt np1West Hollow MS Ms. Gill
╠²
This document provides information about Earth's four spheres: the geosphere, atmosphere, hydrosphere, and biosphere. It defines each sphere and provides some key details about their composition and interactions. The geosphere is the solid Earth, consisting of all interior layers from the surface to the core. The atmosphere is the gaseous envelope surrounding Earth. The hydrosphere includes all liquid and solid water on Earth, such as oceans, lakes, rivers, and glaciers. Finally, the biosphere comprises all living things on Earth, from microorganisms to plants and animals. The document also notes that the spheres interact and influence each other.Time Presentation 



Time Presentation Vj NiroSh
╠²
This document discusses different types of time measurement:
- Sidereal time is based on the motion of stars, while solar time is based on the motion of the sun.
- Local sidereal time is the hour angle of the first point of Aries measured from the local meridian.
- Mean solar time is measured based on the interval between the mean sun's position at the vernal equinox.
- Greenwich mean time is measured from the Greenwich meridian and is also known as universal time. Standard time uses the same time zone to avoid confusion caused by different local times.projection.pptx



projection.pptxAsmitaKanav1
╠²
The document provides an overview of map projections, which are mathematical transformations that convert the spherical coordinates on the Earth's surface to planar coordinates that can be represented on a map. It discusses the different types of projections, including cylindrical, conical, and planar projections. It also covers important concepts like datums, ellipsoids, and how different projections preserve properties like shapes, distances, areas, or directions to varying degrees based on their mathematical properties and construction.Cartography Unit_ 3.pptx



Cartography Unit_ 3.pptxwarkisafile1
╠²
This document provides information on map projections. It defines map projection as a systematic transformation of locations on Earth onto a plane. It discusses the three main types of projections: planar, cylindrical, and conic. Planar projections center on a point and are accurate near the center, cylindrical projections are rolled onto a cylinder and accurate along the equator, and conic projections use a cone and are suited to limited east-west areas near the equator. It also discusses properties like shape, area, direction, and distance distortions that occur in projections and notes no projection is perfect. Common projections like Mercator, UTM, and Robinson are described.Lecture 2.pptx.pdf



Lecture 2.pptx.pdfssuser7386811
╠²
This document provides an overview of satellite communications and orbital mechanics. It discusses the key forces that act on satellites in stable orbits, including centrifugal force and gravitational attraction. Kepler's laws of planetary motion are also summarized. The document outlines different types of satellite orbits, orbital parameters, and how to calculate look angles to track a satellite's position from an earth station. It describes the general architecture of satellite communication systems, including the space segment consisting of the satellite and ground control station, as well as different types of ground terminals.Surveying geodesy ajith sir



Surveying geodesy ajith sirSHAMJITH KM
╠²
The Earth is not a perfect sphere, but is slightly flattened at the poles. The Earth rotates daily on its tilted axis, causing seasons and influencing climate. Parallels of latitude and meridians of longitude form a grid system to locate positions on the Earth's surface. The Earth revolves around the Sun annually in an elliptical orbit, with the seasons resulting from the tilt of its axis of rotation. Precise geodetic coordinates define locations on the reference ellipsoid used to model the oblate spheroid shape of the Earth.3.2.2.a_orbitmechmodeling.pptx



3.2.2.a_orbitmechmodeling.pptxsanthyamuthu1
╠²
Orbital mechanics describes the motion of objects in orbit around other celestial bodies under the influence of gravity. An orbit is a regular, repeating path determined by Kepler's laws of planetary motion. Orbital elements such as eccentricity, semi-major axis, inclination, and others are used to quantitatively describe the size, shape, and orientation of orbits in space. Different types of orbits like low Earth orbit, geostationary orbit, and Molniya orbit are suited for different purposes like Earth observation, communications, and coverage of high latitude regions.More from bandiprathyu (20)
lecture3.ppt cellular system topics are covered



lecture3.ppt cellular system topics are coveredbandiprathyu
╠²
In this ppt you can get the topics related to cellular system.UNIT-I PPT (2).pptx Cell shape analog & Digital



UNIT-I PPT (2).pptx Cell shape analog & Digitalbandiprathyu
╠²
In this ppt Cell shape and analog and digital systems are coveredunit1chapter1-171125094511.pdf cellular mobile radio system



unit1chapter1-171125094511.pdf cellular mobile radio systembandiprathyu
╠²
Cellular mobile radio system topics are covered in this unit.Unit 6 gps.ppt presentation on gps system



Unit 6 gps.ppt presentation on gps systembandiprathyu
╠²
This presentation consists of GPS concepts in satellite communication system.Unit 4 Multiple Access.ppt presentation for Satellite communication



Unit 4 Multiple Access.ppt presentation for Satellite communicationbandiprathyu
╠²
This ppt presentation consists of Multiple access techniques in satellite communication.UHV UNIT-3 is the Harmony in the family and society.pdf



UHV UNIT-3 is the Harmony in the family and society.pdfbandiprathyu
╠²
This unit explains about the harmony in the familiy and societyUNIT-II-is related to HARMONY IN MYSELF in UHV.docx



UNIT-II-is related to HARMONY IN MYSELF in UHV.docxbandiprathyu
╠²
This Unit explains about the Harmony in myself.SATELLITE COMMUNICATIONS UNIT-1 FINAL PPT.pdf



SATELLITE COMMUNICATIONS UNIT-1 FINAL PPT.pdfbandiprathyu
╠²
Satellite communication orbital mechanics.Recently uploaded (20)
Taykon-Kalite belgeleri



Taykon-Kalite belgeleriTAYKON
╠²
Kalite Politikam─▒z
Taykon ├ćelik i├¦in kalite, hayallerinizi bizlerle payla┼¤t─▒─¤─▒n─▒z an ba┼¤lar. Proje ├¦iziminden detaylar─▒n ├¦├Čz├╝m├╝ne, detaylar─▒n ├¦├Čz├╝m├╝nden ├╝retime, ├╝retimden montaja, montajdan teslime hayallerinizin ger├¦ekle┼¤ti─¤ini g├Črd├╝─¤├╝n├╝z ana kadar ge├¦en t├╝m a┼¤amalar─▒, ├¦al─▒┼¤anlar─▒, t├╝m teknik donan─▒m ve ├¦evreyi i├¦ine al─▒r KAL─░TE.Turbocor Product and Technology Review.pdf



Turbocor Product and Technology Review.pdfTotok Sulistiyanto
╠²
High Efficiency Chiller System in HVACImproving Surgical Robot Performance Through Seal Design.pdf



Improving Surgical Robot Performance Through Seal Design.pdfBSEmarketing
╠²
Ever wonder how something as "simple" as a seal can impact surgical robot accuracy and reliability? Take quick a spin through this informative deck today, and use what you've learned to build a better robot tomorrow.Unit 1- Review of Basic Concepts-part 1.pptx



Unit 1- Review of Basic Concepts-part 1.pptxSujataSonawane11
╠²
DS, ADT, Algorithms, Asymptotic Notations are summarized. GE 6B GT Ratcheting Animation- Hemananda Chinara.ppsx



GE 6B GT Ratcheting Animation- Hemananda Chinara.ppsxHemananda Chinara
╠²
GE 6B Gas Turbine Ratcheting Mechanism Animation made by Hemananda Chinara, SIC, CPP, HPL.Designing Flex and Rigid-Flex PCBs to Prevent Failure



Designing Flex and Rigid-Flex PCBs to Prevent FailureEpec Engineered Technologies
╠²
Flex and rigid-flex printed circuit boards (PCBs) can be considered at the basic level some of the most complex PCBs in the industry. With that in mind, itŌĆÖs incredibly easy to make a mistake, to leave something out, or to create a design that was doomed from the start.
Such design failures can end up leading to an eventual failure by delamination, short circuits, damage to the flex portions, and many other things. The easiest way to circumvent these is to start at the beginning, to design with preventing failure in mind rather than trying to fix existing designs to accommodate for problems.
In this webinar, we cover how to design flex and rigid-flex PCBs with failure prevention in mind to save time, money, and headaches, and what failure can look like.
For more information on our flex and rigid-flex PCB solutions, visit https://www.epectec.com/flex.TASK-DECOMPOSITION BASED ANOMALY DETECTION OF MASSIVE AND HIGH-VOLATILITY SES...



TASK-DECOMPOSITION BASED ANOMALY DETECTION OF MASSIVE AND HIGH-VOLATILITY SES...samueljackson3773
╠²
The Science Information Network (SINET) is a Japanese academic backbone network for more than 800
universities and research institutions. The characteristic of SINET traffic is that it is enormous and highly
variableESIT135 Problem Solving Using Python Notes of Unit-2 and Unit-3



ESIT135 Problem Solving Using Python Notes of Unit-2 and Unit-3prasadmutkule1
╠²
ESIT135 Problem Solving Using Python Notes of Unit-2 and Unit-3IoT-based-Electrical-Motor-Fault-Detection-System.pptx



IoT-based-Electrical-Motor-Fault-Detection-System.pptxatharvapardeshi03
╠²
IoT-based-Electrical-Motor-Fault-Detection-System.pptxHelium Boosting & Decanting With Hydro Test Machine



Helium Boosting & Decanting With Hydro Test MachinePaskals Fluid Systems Pvt. Ltd.
╠²
About:
A helium boosting and decanting system is typically used in various industrial applications, particularly in the production and handling of gases, including helium including leak test of reciprocating cylinder. HereŌĆÖs a brief overview of its components and functions:
Components
1. Helium Storage Tanks: High-pressure tanks that store helium@ 150 bars.
2. Boosting Pumps: Designed to boost helium pressure up to 150 bar, ensuring efficient flow throughout the system.
3. Decanting Unit: Separates liquid helium from gas, facilitating decanting at pressures of up to 2 bars.
4. Pressure Regulators: Maintain and control the pressure of helium during transport.
5. Control Valves: automatic control valve is provided for the flow and direction of helium through the system.
6. Piping and Fittings: High-quality, corrosion-resistant materials for safe transport.
Functions
ŌĆó Boosting Pressure: The system boosts helium pressure up to 150 bar for various applications.
ŌĆó Decanting: Safely decants helium, separating liquid from gas at pressures of up to 2 bar.
ŌĆó Safety Measures: Equipped with relief valves and emergency shut-off systems to handle high pressures safely.
ŌĆó Monitoring and Control: Sensors and automated controls monitor pressure and flow rates.
Application:
ŌĆó Cryogenics: Cooling superconducting magnets in MRI machines and particle accelerators.
ŌĆó Welding: Used as a shielding gas in welding processes.
ŌĆó Research: Crucial for various scientific applications, including laboratories and space exploration.
Key Features:
ŌĆó Helium Storage & Boosting System
ŌĆó Decanting System
ŌĆó Pressure Regulation & Monitoring
ŌĆó Valves & Flow Control
ŌĆó Filtration & Safety Components
ŌĆó Structural & Material Specifications
ŌĆó Automation & Electrical Components
Environmental Product Declaration - Uni Bell



Environmental Product Declaration - Uni BellManishPatel169454
╠²
The Uni-Bell PVC Pipe Association (PVCPA) has published the first North American industry-wide environmental product declaration (EPD) for water and sewer piping, and it has been verified by NSF Sustainability, a division of global public health organization NSF International.Data recovery and Digital evidence controls in digital frensics.pdf



Data recovery and Digital evidence controls in digital frensics.pdfAbhijit Bodhe
╠²
This topic contain information about Data recovery and Digital evidence controls in cyber and digital awarenessFailover System in Cloud Computing System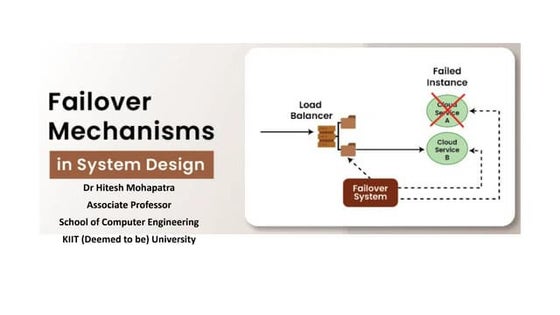



Failover System in Cloud Computing SystemHitesh Mohapatra
╠²
Uses established clustering technologies for redundancy
Boosts availability and reliability of IT resources
Automatically transitions to standby instances when active resources become unavailable
Protects mission-critical software and reusable services from single points of failure
Can cover multiple geographical areas
Hosts redundant implementations of the same IT resource at each location
Relies on resource replication for monitoring defects and unavailability conditionsSppu engineering artificial intelligence and data science semester 6th Artif...



Sppu engineering artificial intelligence and data science semester 6th Artif...pawaletrupti434
╠²
Sppu University Third year AI&DS Artificial Neural Network unit 1AI-Powered Power Converter Design Workflow.pdf



AI-Powered Power Converter Design Workflow.pdfAleksandr Terlo
╠²
Blending human expertise with AI-driven optimization for efficient power converter design.Unit-2 Look Angle Determination.ppt inSC
- 1. Unit-II Look Angle Determination Mrs B.Prathyusha Asst.Professor Department of ECE
- 2. Reference Satellite Communications, ŌĆØ Timothy Pratt ,Jeremy Allnutt ŌĆ£, Third Edition, Wiley 2020.
- 3. Learning Outcome ŌĆó Students will be able to determine the look angles of a satellite.
- 4. Contents ŌĆó Look Angle Determination : Azimuthal Angle, Elevation Angle ŌĆó Subsatellite Point :Nadir Direction, Zenith Direction ŌĆó Azimuthal Angle Measurement ŌĆó Elevation Angle Measurement ŌĆó Visibility Test
- 5. Look Angle Determination ŌĆó The coordinates to which an earth station antenna must be pointed to communicate with a satellite are called the look angles. ŌĆó These are most commonly expressed as azimuth (Az) and elevation (El), although other pairs exist. ŌĆó Azimuth Angle ŌĆó Elevation Angle
- 6. ŌĆó Generally, the values of these angles change for non-geostationary orbits. Whereas, the values of these angles donŌĆÖt change for geostationary orbits. Because, the satellites present in geostationary orbits appear stationary with respect to earth. ŌĆó These two angles are helpful in order to point at the satellite directly from the earth station antenna. So, the maximum gain of the earth station antenna can be directed at satellite.
- 7. ŌĆó We can calculate the look angles of geostationary orbit by using longitude & latitude of earth station and position of satellite orbit. ŌĆó Azimuth Angle ŌĆó The angle between local horizontal plane and the plane passing through earth station, satellite and center of earth is called as azimuth angle. ŌĆó Elevation Angle ŌĆó The angle between vertical plane and line pointing to satellite is known as Elevation angle. Vertical plane is nothing but the plane, which is perpendicular to horizontal plane.
- 8. Azimuth is measured eastward (clockwise) from geographic north to the projection of the satellite path on a (locally) horizontal plane at the earth station. Elevation is the angle measured upward from the local horizontal plane at the earth station to the satellite path.
- 9. ŌĆó Navigation around the earthŌĆÖs oceans became more precise when the surface of the globe was divided up into a grid-like structure of orthogonal lines: latitude and longitude. ŌĆó Latitude is the angular distance, measured in degrees, north or south of the equator and longitude is the angular distance, measured in degrees, from a given reference longitudinal line. ŌĆó England drew its reference zero longitude through Greenwich, a town close to London, England, and France, not surprisingly, drew its reference longitude through Paris, France.
- 10. ŌĆó When GEO satellite systems are registered in Geneva, their (subsatellite) location over the equator is given in degrees east to avoid confusion. Thus, the INTELSAT primary location in the Indian Ocean is registered at 60┬░E and the primary location in the Atlantic Ocean at 335.5┬░E (not 24.5┬░W). ŌĆó Earth stations that communicate with satellites are described in terms of their geographic latitude and longitude when developing the pointing coordinates that earth station must use to track the apparent motion of the satellite.
- 11. The Subsatellite Point ŌĆó The subsatellite point is the location on the surface of the earth that lies directly between the satellite and the center of the earth. ŌĆó It is the nadir pointing direction from the satellite and, for a satellite in an equatorial orbit, it will always be located on the equator. ŌĆó Since geostationary satellites are in equatorial orbits and are designed to stay stationary over the earth, it is usual to give their orbital location in terms of their subsatellite point.
- 12. ŌĆó To an observer of a satellite standing at the subsatellite point, the satellite will appear to be directly overhead, in the zenith direction from the observing location. ŌĆó The zenith and nadir paths are therefore in opposite directions along the same path ŌĆó Designers of satellite antennas reference the pointing direction of the satelliteŌĆÖs antenna beams to the nadir direction.
- 13. ŌĆó The communications coverage region on the earth from a satellite is defined by angles measured from nadir at the satellite to the edges of the coverage. ŌĆó Earth station antenna designers, however, do not reference their pointing direction to zenith. ŌĆó As noted earlier, they use the local horizontal plane at the earth station to define elevation angle and geographical compass points to define azimuth angle, thus giving the two look angles for the earth station antenna toward the satellite (Az, El).
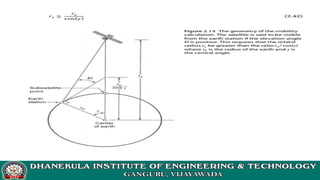
- 15. ŌĆó Figure shows the geometry of the elevation angle calculation. ŌĆó rs is the vector from the center of the earth to the satellite ŌĆó re is the vector from the center of the earth to the earth station ŌĆó d is the vector from the earth station to the satellite ŌĆó These three vectors lie in the same plane and form a triangle.
- 16. ŌĆó The central angle ╬│ measured between re and rs is the angle between the earth station and the satellite ŌĆó Žł is the angle (within the triangle) measured from re to d. ŌĆó Defined so that it is non-negative, ╬│ is related to the earth station north latitude Le (i.e., Le is the number of degrees in latitude that the earth station is north from the equator) and west longitude le (i.e., le is the number of degrees in longitude that the earth station is west from the Greenwich meridian) and the subsatellite point at north latitude Ls and west longitude ls by
- 18. Azimuth Angle Calculation ŌĆó Since the earth station, the center of the earth, the satellite, and the subsatellite point all lie in the same plane, the azimuth angle Az from the earth station to the satellite is the same as the azimuth from the earth station to the subsatellite point. ŌĆó This is more difficult to compute than the elevation angle because the exact geometry involved depends on whether the subsatellite point is east or west of the earth station, and in which of the hemispheres the earth station and the subsatellite point are located.
- 21. Visibility Test For a satellite to be visible from an earth station, its elevation angle El must be above some minimum value, which is at least 0┬░. A positive or zero elevation angle requires that
- 25. Any Queries ? Thank You
















