Logarithma
?Download as PPTX, PDF?
0 likes?1,465 views
This document provides information about logarithms including: - The definition of logarithms and their relationship to exponents - Examples of evaluating logarithmic expressions - Properties of logarithms including product, quotient, and power properties - How to expand and condense logarithmic expressions using these properties - How to solve logarithmic equations by using the properties of logarithms.
Logarithma
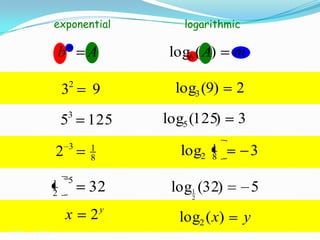
- 2. LOGARITHMS exponential logarithmic m b A logb ( A) m b>0 A>0 Jeff Bivin -- LZHS
- 3. exponential logarithmic m b A logb ( A) m 2 3 9 log3 (9) 2 53 125 log5 (125) 3 3 1 1 2 8 log2 8 3 1 5 2 32 log1 (32) 5 2 y x 2 log2 ( x) y Jeff Bivin -- LZHS
- 4. Evaluate log5 (25) u u 5 25 u 2 5 5 u 2 log5 (25) 2 Jeff Bivin -- LZHS
- 5. Evaluate log3 (81) u u 3 81 u 4 3 3 u 4 log3 (81) 4 Jeff Bivin -- LZHS
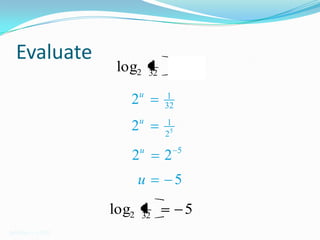
- 6. Evaluate 1 log2 32 u u 1 2 32 u 1 2 25 2u 2 5 u 5 1 log2 32 5 Jeff Bivin -- LZHS
- 7. Try this!! log7 (7) u u 7 7 u 1 7 7 u 1 log7 (7) 1 Jeff Bivin -- LZHS
- 8. Try this!! Solve for x logx(32) = 5 x5 = 32 x5 = 25 x = 2 Jeff Bivin -- LZHS
- 9. Try this!! loga (1) ? 0 a 1 loga (1) 0 Jeff Bivin -- LZHS
- 10. Try this!! loga (a) ? 1 a a loga (a) 1 Jeff Bivin -- LZHS
- 11. Special Logarithms log10 a log a loge a ln a
- 12. Properties of Logarithms ? Product Property ? Quotient Property ? Power Property Jeff Bivin -- LZHS
- 13. Product Property m n m n a a a multiplication addition logb (m n) logb (m) logb (n) multiplication addition Jeff Bivin -- LZHS
- 14. Product Property log2 (16 4) log2 (16) log2 (4) 4 2 4 2 log2 (2 2 ) log2 (2 ) log2 (2 ) 6 log2 (2 ) 4 2 6 6 Jeff Bivin -- LZHS
- 15. Quotient Property m a m n division n a subtraction a m log ( ) b n logb (m) logb (n) division subtraction Jeff Bivin -- LZHS
- 16. Quotient Property 32 log2 4 log2 (32) log2 (4) 5 2 log2 8 log2 (2 ) log2 (2 ) 3 log2 (2 ) 5 2 3 3 Jeff Bivin -- LZHS
- 17. Power Property m n mn a a p logb(m p ) logb(mp ) = p?logb(m) Jeff Bivin -- LZHS
- 18. Power Property 7 log2 2 7 log2 (2) 7 71 7 7 Jeff Bivin -- LZHS
- 19. Power Property n log a a n n.log a ( a ) n.1 n Jeff Bivin -- LZHS
- 20. Change of Base Formula logb x loga x logb a log x log a x log a Jeff Bivin -- LZHS
- 21. log b 1 1 log a b log a log a logb a log b log b log c log c log a b.logb c . log a c log a log b log a n n log b n.log b n log am b m .log a b log a m.log a m Jeff Bivin -- LZHS
- 22. Expand 3 2 log5 ( x y ) 3 2 product property log5 ( x ) log5 ( y ) power property 3 log5 ( x) 2 log5 ( y) Jeff Bivin -- LZHS
- 23. Expand x7 log 5 y2 z5 7 2 5 quotient property log5 ( x ) log5 ( y z ) product property log5 ( x )7 2 log5 ( y ) 5 log5 ( z ) distributive property log5 ( x )7 2 log5 ( y ) 5 log5 ( z ) power property 7 log5 ( x) 2 log5 ( y) 5 log5 ( z) Jeff Bivin -- LZHS
- 24. Condense 5 log3 x 6 log3 y 2 log3 z power property log3 x 5 log3 y 6 log3 z 2 product property log3 x y 5 6 log3 z 2 x5 y 6 quotient property log 3 z2 Jeff Bivin -- LZHS
- 25. Condense 1 2 log10 x 2 log10 y 4 log10 z 1 2 4 Power property log10 x 2 log10 y log10 z 1 2 4 group / factor log10 x 2 log10 y log10 z 1 product property 2 4 log10 x 2 log10 y z 1 quotient property log10 x2 log x y2z4 10 y 2 z 4 Jeff Bivin -- LZHS
- 26. Logarithm Equations Properties log a B log a C B C Jeff Bivin -- LZHS
- 27. Solve for x log3 3x 9 log3 x 3 3x 9 x 3 2x 12 x 6 Jeff Bivin -- LZHS
- 28. Solve for x log3 3x 9 log3 x 3 check x 6 log3 3(6) 9 log3 6 3 log3 18 9 log3 6 3 log3 9 log3 9 checks! Jeff Bivin -- LZHS
- 29. Solve for x log3 3x 9 log3 x 3 3x 9 x 3 2x 12 x 6 6 Jeff Bivin -- LZHS
- 30. Solve for x log4 7 log4 n 2 log4 6n log4 7(n 2) log4 6n 7n 14 6n n 14 Jeff Bivin -- LZHS
- 31. Solve for x log4 7 log4 n 2 log4 6n check n 14 log4 7 log4 14 2 log4 6(14) log4 7 log4 12 log4 84 log4 7(12) log4 84 log4 84 log4 84 checks! Jeff Bivin -- LZHS
- 32. Solve for x log4 7 log4 n 2 log4 6n log4 7(n 2) log4 6n 7n 14 6n n 14 14 Jeff Bivin -- LZHS
- 33. Solve for x log2 x 1 log2 x 1 3 3 log 2 ( x 1)( x 1) log 2 2 3 ( x 1)( x 1) 2 2 x 1 8 2 x 9 x 3 Jeff Bivin -- LZHS
- 34. Solve for x log2 x 1 log2 x 1 3 check x 3 check x 3 log2 3 1 log2 3 1 3 log2 3 1 log2 3 1 3 log2 4 log2 2 3 log2 2 log2 4 3 2 1 3 fails 3 3 The argument checks! must be positive Jeff Bivin -- LZHS
- 35. Solve for x log2 x 1 log2 x 1 3 log2 ( x 1)(x 1) 3 3 2 ( x 1)(x 1) 2 8 x 1 2 9 x 3 x 3 Jeff Bivin -- LZHS
- 36. x x 3 3 27 3 3 x 3 x x 2 5 49 5 7 ????????
- 37. Solve exponential equation with logarithms x x 2 5 49 5 7 ???????? log 5 x log 7 2 x.log 5 2.log 7 log 7 x 2. log 5 x 2.log 5 7
- 38. Solve for x 4x 2 log 5 log 7 (4 x) log(5) (2) log(7) 4 log(5) 4 log(5) 2 log( 7 ) x 4 log(5 ) log(7 2 ) x log(54 ) x log 625 (49) Jeff Bivin -- LZHS
- 39. Solve for x x 2 x 1 log 3 log 5 ( x 2) log(3) ( x 1) log(5) x log(3) 2 log(3) x log(5) 1log(5) x log(3) x log(5) log(5) 2 log(3) x log(3) log(5) log(5) 2 log(3) 5 log( ) 5 x 9 3 x log 3 ( ) log( ) ( ) 9 5 5 Jeff Bivin -- LZHS
- 40. Try this 3x 1 5 7 2 5 3x 1 log 7 log 2 5 log(7 ) (3x 1) log(2) 5 log( 7 ) 3x log(2) 1 log(2) 5 log(7 ) log(2) 3x log(2) log( 10 ) log(8) 7 x 10 log ( ) Jeff Bivin -- LZHS 8 7 x
- 41. Try this 3x 2 ln 15 ln e ln(15) 1 (3x 2) ln(e) ln(15) 3x 2 ln(15) 2 3x ln(15) 2 3 x Jeff Bivin -- LZHS